Skip to page content Home     Posts     CV     Contact     People

The Gamma and Lambert-W Functions

2017 June 21

Find the values of the following integrals, where $W(x)$ denotes the inverse function of $y=xe^x$ from $0$ to $\infty$: $$\int_0^1 W(x)dx$$ $$\int_0^{e} W(x)^2dx$$ $$\int_0^\infty W(e^{-x})dx$$ $$\int_0^\infty \frac{W(x)}{x\sqrt{x}}dx$$ $$\int_0^\infty W\bigg(\frac{1}{x^2}\bigg)dx$$

I will be devoting this post to two very interesting and related functions: the Gamma Function $\Gamma(z)$ and the Lambert-W Function $W(z)$.

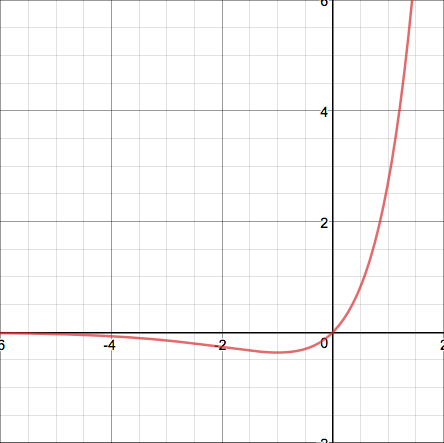
I will start off with the basics of the Lambert-W function. This function is taken to be the inverse function of $y=xe^x$. Here are two graphs, one of $y=xe^x$ (in red), and one of the Lambert-W function (in blue).

As you can see, $y=xe^x$ is not injective and should not have an inverse, but because only a small branch of it is non-injective, it can be useful to treat is as if it had an inverse anyways. This is what the Lambert-W function is for. That function cannot be inverted anyways using elementary functions, so the Lambert-W function is what we treat as its inverse. However, since it is not actually injective, the Lambert-W function has two branches: the lower branch $W_{-1}$ and the upper branch $W_0$. The point at which the function changes between these two branches is at the point corresponding to the minimum of $y=xe^x$, the point $(-\frac{1}{e},-1)$ on the Lambert-W function. Because it is defined as the inverse of $y=xe^x$, it has the properties $$W(xe^x)=x$$ $$W(x)e^{W(x)}=x$$

The value $W(1)$, or the unique solution to the equation $$xe^x=1$$ is called the omega constant $\Omega$ and is about $0.5671$. We will be using it in some of our later integral problems.

This function can be used to "solve" many new types of equations. For example, consider the equation $$e^x=3x$$ This equation can be solved using the Lambert-W function in the following way: $$e^x=3x$$ $$1=3xe^{-x}$$ $$-\frac{1}{3}=-xe^{-x}$$ $$W\bigg(-\frac{1}{3}\bigg)=W(-xe^{-x})$$ $$W\bigg(-\frac{1}{3}\bigg)=-x$$ $$x=-W\bigg(-\frac{1}{3}\bigg)$$ There are two possible values satisfying this, since both branches of the Lambert-W function exist at $x=-\frac{1}{3}$.

Here's another example: $$6\cdot 2^{2x}=x$$ This can be solved in the following way: $$6\cdot 2^{2x}=x$$ $$6\cdot e^{\ln(2^{2x})}=x$$ $$6\cdot e^{2x\ln(2)}=x$$ $$6=e^{-2x\ln(2)}x$$ $$-12\ln(2)=-2x\ln(2)e^{-2x\ln(2)}$$ $$W(-12\ln(2))=W(-2x\ln(2)e^{-2x\ln(2)})$$ $$W(-12\ln(2))=-2x\ln(2)$$ $$x=-\frac{W(-12\ln(2))}{2\ln(2)}$$

The derivative of the Lambert-W function is given by $$W'(x)=\frac{1}{x}\frac{W(x)}{W(x)+1}$$ I will not go into the details of the derivation of this formula, because it can be attained easily using the formula for the derivative of the inverse of a function, in this case the function $f(x)=xe^x$. Furthermore, its antiderivative is $$\int W(x) dx=\frac{x(W(x)^2-W(x)+1)}{W(x)}+C$$ which can also be obtained with the use of a formula.

We will now derive two identities of the Lambert-W function that we will now prove that may become helpful later. The first is the sum identity $$W(a)+W(b)=W\bigg(\frac{ab}{W(a)}+\frac{ab}{W(b)}\bigg)$$ and the second is the product identity $$aW(b)=W\bigg(\frac{ab^a}{W(b)^{a-1}}\bigg)$$ Here is the derivation of the first identity: $$W(a)+W(b)$$ $$=W\bigg((W(a)+W(b))e^{W(a)+W(b)}\bigg)$$ $$=W\bigg(W(a)e^{W(a)}e^{W(b)}+W(b)e^{W(b)}e^{W(a)}\bigg)$$ $$=W\bigg(ae^{W(b)}+be^{W(a)}\bigg)$$ $$=W\bigg(\frac{ab}{W(b)}+\frac{ab}{W(a)}\bigg)$$ $$=W\bigg(\frac{ab}{W(a)}+\frac{ab}{W(b)}\bigg)$$ And here is the derivation of the second: $$aW(b)$$ $$=W\bigg(aW(b)e^{aW(b)}\bigg)$$ $$=W\bigg(a\cdot W(b)e^{W(b)}\cdot e^{(a-1)W(b)}\bigg)$$ $$=W\bigg(abe^{(a-1)W(b)}\bigg)$$ $$=W\bigg(ab\big(e^{W(b)}\big)^{a-1}\bigg)$$ $$=W\bigg(ab\bigg(\frac{b}{W(b)}\bigg)^{a-1}\bigg)$$ $$=W\bigg(\frac{ab^a}{W(b)^{a-1}}\bigg)$$

Before we move into the "good stuff", it would be best to introduce the Gamma Function, as it can save a lot of time when evaluating some of the integrals that we will later evaluate.

Basically, the Gamma Function is an extension of the factorial function to non-natural numbers. It has the property $$\Gamma(n)=(n-1)!, \forall n\in\mathbb N$$ So it is essentially the factorial function, translated one unit. It is defined as $$\Gamma(z)=\int_0^\infty x^{z-1}e^{-x}dx$$ Its relation to the factorial function can be proven using induction. First we must show that $\Gamma(1)=1$: $$\Gamma(1)=\int_0^\infty x^{1-1}e^{-x}dx$$ $$\Gamma(1)=\int_0^\infty e^{-x}dx$$ $$\Gamma(1)=1$$

Wonderful. Now we must begin the inductive part of the proof. $$\Gamma(z)=\int_0^\infty x^{z-1}e^{-x}dx$$ If we use integration by parts, we can observe that $$\int x^{z-1}e^{-x}dx=-x^{z-1}e^{-x}+(z-1)\int x^{z-2}e^{-x}dx$$ Which means that $$\Gamma(z)=(z-1)\int_0^\infty x^{z-2}e^{-x}dx$$ $$\Gamma(z)=(z-1)\Gamma(z-1)$$ Which completes our inductive proof.

One thing that is helpful when working with the Gamma Function is knowledge of the Gaussian Integral; that is, the integral $$\int_{-\infty}^\infty e^{-x^2}dx=\sqrt{\pi}$$ This integral comes up often when evaluating particular values of the Gamma Function. For example, look what happens when we try to evaluate $\Gamma\bigg(\frac{1}{2}\bigg)$: $$\Gamma\bigg(\frac{1}{2}\bigg)=\int_0^\infty x^{-\frac{1}{2}}e^{-x}dx$$ If we make the substitution $x \to y^2$, this integral turns into $$\Gamma\bigg(\frac{1}{2}\bigg)=\int_0^\infty 2y(y^2)^{-\frac{1}{2}}e^{-y^2}dx$$ $$\Gamma\bigg(\frac{1}{2}\bigg)=2\int_0^\infty e^{-y^2}dx$$ $$\Gamma\bigg(\frac{1}{2}\bigg)=\int_{-\infty}^\infty e^{-y^2}dx$$ Which is just the same as the Gaussian Integral, and so $$\Gamma\bigg(\frac{1}{2}\bigg)=\sqrt{\pi}$$

I'll spare you the derivations of the first couple values of this type of the Gamma Function, as they each involve a lot of integration by parts and are very repetitive. Here they are: $$\Gamma\bigg(\frac{1}{2}\bigg)=\sqrt{\pi}$$ $$\Gamma\bigg(\frac{3}{2}\bigg)=\frac{1}{2}\sqrt{\pi}$$ $$\Gamma\bigg(\frac{5}{2}\bigg)=\frac{3}{4}\sqrt{\pi}$$ $$\Gamma\bigg(\frac{7}{2}\bigg)=\frac{15}{8}\sqrt{\pi}$$

Are you noticing a pattern? $$\Gamma\bigg(\frac{1}{2}\bigg)=\frac{1}{2^0}\sqrt{\pi}$$ $$\Gamma\bigg(\frac{3}{2}\bigg)=\frac{1}{2^1}\sqrt{\pi}$$ $$\Gamma\bigg(\frac{5}{2}\bigg)=\frac{1\cdot3}{2^2}\sqrt{\pi}$$ $$\Gamma\bigg(\frac{7}{2}\bigg)=\frac{1\cdot3\cdot5}{2^3}\sqrt{\pi}$$

Do you see it? This can lead us to conjecture that $$\Gamma\bigg(\frac{2n+1}{2}\bigg)=\frac{(2n-1)!!}{2^n}, \forall n \in \mathbb N$$

This can be proven easily using induction. This proof is also inductive and also uses integration by parts. The first step is recalling that $\Gamma(\frac{1}{2})$ is equal to $\sqrt{\pi}$. Then we can begin our inductive step. By definition, $$\Gamma\bigg(\frac{2n+1}{2}\bigg)=\int_0^\infty x^{\frac{2n-1}{2}}e^{-x}dx$$ And if we use integration by parts, we notice that $$\int x^{\frac{2n-1}{2}}e^{-x}dx=-x^{\frac{2n-1}{2}}e^{-x} + \frac{2n-1}{2}\int x^{\frac{2n-3}{2}}e^{-x}dx$$ Meaning that $$\Gamma\bigg(\frac{2n+1}{2}\bigg)=\frac{2n-1}{2}\int_0^\infty x^{\frac{2n-3}{2}}e^{-x}dx$$ $$\Gamma\bigg(\frac{2n+1}{2}\bigg)=\frac{2n-1}{2}\Gamma\bigg(\frac{2n-1}{2}\bigg)$$ Which completes the induction step of our proof.

It can be proven similarly that $$\Gamma\bigg(\frac{kn+1}{k}\bigg)=\frac{(kn-k+1)!_k}{k^n}, \forall n,k \in \mathbb N$$ where $!_k$ represents the kth factorial (that is, $!_2$ is $!!$, $!_3$ is $!!!$, and so on). This fact will be useful to us later on.

One final notable formula regarding the Gamma Function is its reflection formula, derived by Euler: $$\Gamma(z)\Gamma(1-z)=\frac{\pi}{\sin \pi z}$$

However, we will not use it much, and so we will not derive it here. Perhaps in a later post that is focused solely on the Gamma Function.

Before we start the integrals, let me remind you of the definite integral property $$\int_a^b f(x)dx=\int_{g^{-1}(a)}^{g^{-1}(b)} g'(x)(f \circ g)(x) dx$$ Because we will be using it a lot in the upcoming problems.

First off, we will tackle the least intimidating of the integrals: $$\int_0^1 W(x)dx$$ It seems impossible at first to integrate over a function that cannot even be expressed using other elementary functions. However, we can use the trick that I just mentioned, along with the fact that $W(x)$ is defined as the inverse of $xe^x$. Let's use the trick with $g(x)=xe^x$. Then we get $$\int_{W(0)}^{W(1)} W(xe^x)\cdot\frac{d}{dx}(xe^x)dx$$ $W(0)$ is $0$ and $W(1)$ is the omega constant, so we can now simplify the integral and the rest of the bounds: $$\int_{0}^{\Omega} x(xe^x+e^x)dx$$ $$\int_{0}^{\Omega} x^2e^xdx+\int_0^{\Omega} xe^xdx$$ Using integration by parts, we can reduce this to $$\Omega^2 e^{\Omega}-2\int_{0}^{\Omega} xe^xdx+\int_0^{\Omega} xe^xdx$$ $$\Omega^2 e^{\Omega}-\int_0^{\Omega} xe^xdx$$ $$\Omega^2 e^{\Omega}-(\Omega e^\Omega-e^\Omega+e^0)$$ $$\Omega^2 e^{\Omega}-\Omega e^\Omega+e^\Omega-e^0$$ Remember, since $\Omega=W(1)$, we can simplify $\Omega e^\Omega$ to $1$: $$\Omega-1+e^\Omega-1$$ $$\Omega+e^\Omega-2$$ Furthermore, since $\Omega e^\Omega=1$, then $e^\Omega=\frac{1}{\Omega}$, so our simplified answer is $$\Omega+\frac{1}{\Omega}-2$$ and so $$\int_0^1 W(x)dx=\Omega+\frac{1}{\Omega}-2$$

On to the next one: $$\int_0^{e} W(x)^2dx$$ Let us again use our "trick" with $g(x)=xe^x$: $$\int_0^{1} x^2(xe^x+e^x)dx$$ $$\int_0^{1} x^3e^xdx+\int_0^1 xe^xdx$$ Now the integral can be solved readily by using integration by parts over and over again. I'll spare you the details: $$\int_0^{e} W(x)^2dx=4-e$$

Now for the integral $$\int_0^\infty W(e^{-x})dx$$ This time we can use $g(x)=-\ln(xe^x)=-\ln(x)-x$ to get the much easier integral $$\int_{\Omega}^0 x\cdot -(\frac{1}{x}+1)dx$$ $$\int_{0}^\Omega (x+1)dx$$ $$\frac{\Omega^2}{2}+\Omega$$ and so $$\int_0^\infty W(e^{-x})dx=\frac{\Omega^2}{2}+\Omega$$

Next up is $$\int_0^\infty \frac{W(x)}{x\sqrt{x}}dx$$ Let us once again use $g(x)=xe^x$ to get $$\int_0^\infty \frac{x(xe^x+e^x)}{xe^x\sqrt{xe^x}}dx$$ $$\int_0^\infty \frac{xe^x(x+1)}{xe^x\sqrt{xe^x}}dx$$ $$\int_0^\infty \frac{x+1}{\sqrt{xe^x}}dx$$ $$\int_0^\infty (x+1)(x^{-\frac{1}{2}}e^{-\frac{1}{2}x})dx$$ $$\int_0^\infty x^{\frac{1}{2}}e^{-\frac{1}{2}x}dx+\int_0^\infty x^{-\frac{1}{2}}e^{-\frac{1}{2}x}dx$$ Now we can recognize the relevance of the gamma function. If we use $g(x)=2x$ then we get $$2\sqrt{2}\int_0^\infty x^{\frac{1}{2}}e^{-x}dx+\sqrt{2}\int_0^\infty x^{-\frac{1}{2}}e^{-\frac{1}{2}x}dx$$ $$2\sqrt{2}\Gamma\bigg(\frac{3}{2}\bigg)+\sqrt{2}\Gamma\bigg(\frac{1}{2}\bigg)$$ and, since we have already obtained these values for the Gamma Function, we have $$2\sqrt{2}\frac{\sqrt{\pi}}{2}+\sqrt{2}\sqrt{\pi}$$ $$2\sqrt{2\pi}$$ and so $$\int_0^\infty \frac{W(x)}{x\sqrt{x}}dx=2\sqrt{2\pi}$$

Now for the final integral: $$\int_0^\infty W\bigg(\frac{1}{x^2}\bigg)dx$$ First we will use $g(x)=\sqrt{\frac{1}{x}e^{-x}}$, which gives us $$\frac{1}{2}\int_0^\infty x\cdot\bigg(x^{-\frac{1}{2}}e^{-\frac{1}{2}x}+x^{-\frac{3}{2}}e^{-\frac{1}{2}x}\bigg)dx$$ $$\frac{1}{2}\int_0^\infty x^{\frac{1}{2}}e^{-\frac{1}{2}x}dx+\frac{1}{2}\int_0^\infty x^{-\frac{1}{2}}e^{-\frac{1}{2}x}dx$$ Now let us use $g(x)=2x$: $$\frac{1}{2}2\sqrt{2}\int_0^\infty x^{\frac{1}{2}}e^{-x}dx+\frac{1}{2}\sqrt{2}\int_0^\infty x^{-\frac{1}{2}}e^{-x}dx$$ $$\sqrt{2}\Gamma\bigg(\frac{3}{2}\bigg)+\frac{1}{\sqrt{2}}\Gamma\bigg(\frac{1}{2}\bigg)$$ $$\sqrt{2}\frac{\sqrt{\pi}}{2}+\frac{1}{\sqrt{2}}\sqrt{\pi}$$ $$\sqrt{2\pi}$$ and so $$\int_0^\infty W\bigg(\frac{1}{x^2}\bigg)dx=\sqrt{2\pi}$$

And that concludes this blog post!